1D F-Area Full Geochemistry#
Overview and Capabilities tested#
This test example performs the simulation of a complex geochemical network in a simple 1D flow domain, combining reaction types described separately in 1D Tritium first-order decay, 1D Calcite dissolution, and 1D Ion exchange. The reaction network is based on the Savannah River Site F-Area geochemistry. This example tests the following capabilities:
1D flow
1D advective transport
Geochemical reactions
Aqueous complexation reactions (equilibrium)
Radioactive decay
Mineral dissolution
Ion exchange
Surface complexation
For details on this test, see About.
Background#
This problem intends to demonstrate the ASCEM capability of modeling complex geochemical reactions and contaminant transport. The model is based on the one developed at the Savannah River Site F-Area for predicting the uranium and acidic plume migration in the groundwater [BWS+13].
The SRS is located in south-central South Carolina, near Aiken, approximately 100 miles from the Atlantic Coast. The SRS F-Area seepage basins received approximately 7.1 billion litters of acidic, low-level waste solutions from 1950 through 1989 [MLD12]. After the basins were closed and capped in 1991, the site has been under active remediation, including the pump-and-treat and hybrid funnel-and-gate system.
The highly acidic plume including many radionuclides (e.g., uranium isotopes, strontium-90, iodine-129, technetium, and tritium) is developed from the basins to downgradient in groundwater. The concentration of U-238 and pH are of the current main interest at the site. Understanding and predicting the plume mobility and its fate requires modeling complex geochemistry, since pH is influenced by mineral dissolution and precipitation, and the uranium mobility is greatly influenced by pH.
Model#
Flow and transport#
See the 1D Conservative tracer transport example.
Geochemistry#
The primary geochemical system consists of 13 reactive chemical components and 8 minerals [BWS+13]. A detailed list of reactions and geochemical parameters is included in the tables below. Further detail can be found in [FHG+12].
The geochemical processes include:
Equilibrium aqueous complexation (Table 1)
Mineral adsorption/desorption (nonelectrostatic surface complexation) (Table 2)
Equilibrium ion exchange (Table 2)
Kinetically controlled mineral dissolution and precipitation (Table 3)
The sorption model is based on a single-site equilibrium, pH-dependent surface complexation model, which provides the principal control on the uranium migration rate. The nonelectrostatic model used here is applied to the bulk sediment rather than to specific pure mineral phases that serve as sorbents in the conventional electrostatic model. Additionally, the nonelectrostatic model does not assume the presence of well-defined mineral phases [DCKF98]. An ion exchange model includes reactions involving the major cations (\(Ca^{2+}\), \(Na{+}\), and \(Al^{3+}\), along with \(H^+\)) and provides primary pH buffering along with the mineral reactions.
Table 1. Aqueous complexes.
Reaction |
logK (25ºC) |
|---|---|
\(\ce{OH^- <=> H2O - H^+}\) |
13.99 |
\(\ce{AlOH^{2+} <=> H2O - H^+ + Al^{3+}}\) |
4.96 |
\(\ce{Al(OH)2^+ <=> 2 H2O -2 H^+ + Al^{3+}}\) |
10.59 |
\(\ce{Al(OH)3(aq) <=> 3 H2O -3 H^+ + Al^{3+}}\) |
16.16 |
\(\ce{Al(OH)4^- <=> 4 H2O -4 H^+ + Al^{3+}}\) |
22.88 |
\(\ce{CaOH^+ <=> H2O - H^+ + Ca^{2+}}\) |
12.85 |
\(\ce{CaHCO3^+ <=> H2O - H^+ + Ca^{2+} + CO2(aq)}\) |
5.3 |
\(\ce{CaCO3(aq) <=> H2O -2 H^+ + Ca^{2+} + CO2(aq)}\) |
13.35 |
\(\ce{CO3^{2-} <=> H2O -2 H^+ + CO2(aq)}\) |
16.67 |
\(\ce{HCO3^- <=> H2O - H+ + CO2(aq)}\) |
6.34 |
\(\ce{NaCO3^- <=> H2O -2 H^+ + CO2(aq) + Na^+}\) |
16.16 |
\(\ce{NaHCO3(aq) <=> H2O - H^+ + CO2(aq) + Na^+}\) |
6.19 |
\(\ce{NaOH(aq) <=> H2O - H^+ + Na^+}\) |
14.78 |
\(\ce{MgCO3(aq) <=> H2O -2 H^+ + CO2(aq) + Mg^{2+}}\) |
13.69 |
\(\ce{MgOH^+ <=> H2O - H^+ + Mg^{2+}}\) |
11.79 |
\(\ce{MgHCO3^+ <=> H2O - H^+ + CO2(aq) + Mg^{2+}}\) |
5.31 |
\(\ce{(UO2)2(OH)2^{2+} <=> 2 H2O -2 H^+ +2 UO2^{2+}}\) |
5.63 |
\(\ce{(UO2)2CO3(OH)3^- <=> 4 H2O -5 H^+ + CO2(aq) + 2 UO2^{2+}}\) |
17.57 |
\(\ce{(UO2)2OH^{3+} <=> H2O -1 H^+ + 2 UO2^{2+}}\) |
2.71 |
\(\ce{(UO2)3(CO3)6^{6-} <=> 6 H2O -12 H^+ + 6 CO2(aq) + 3 UO2^{2+}}\) |
46.13 |
\(\ce{(UO2)3(OH)4^{2+} <=> 4 H2O -4 H^+ + 3 UO2^{2+}}\) |
11.93 |
\(\ce{UO2(OH)4^{2-} <=> 4 H2O -4 H^+ + UO2^{2+}}\) |
33.03 |
\(\ce{(UO2)3(OH)5^+ <=> 5 H2O -5 H^+ + 3 UO2^{2+}}\) |
15.59 |
\(\ce{(UO2)3(OH)7^- <=> 7 H2O -7 H^+ + 3 UO2^{2}}\) |
31.05 |
\(\ce{(UO2)3O(OH)2(HCO3)^+ <=> 4 H2O -5 H^+ + CO2(aq) + 3 UO2^{2+}}\) |
16.06 |
\(\ce{(UO2)4(OH)7^+ <=> 7 H2O -7 H^+ + 4 UO2^{2+}}\) |
21.95 |
\(\ce{UO2NO3^+ <=> NO3^- + UO2^{2+}}\) |
-0.28 |
\(\ce{UO2OH^+ <=> H2O -1 H^+ + 1 UO2^{2+}}\) |
5.21 |
\(\ce{UO2(OH)2(aq) <=> 2 H2O -2 H^+ + UO2^{2+}}\) |
10.31 |
\(\ce{UO2(OH)3^- <=> 3 H2O -3 H^+ + UO2^{2+}}\) |
19.22 |
\(\ce{UO2CO3(aq) <=> H2O -2 H^+ + CO2(aq) + UO2^{2+}}\) |
7.01 |
\(\ce{UO2(CO3)2^{2-} <=> 2 H2O -4 H^+ + 2 CO2(aq) + UO2^{2+}}\) |
16.44 |
\(\ce{UO2(CO3)3^{4-} <=> 3 H2O -6 H^+ + 3 CO2(aq) + UO2^{2+}}\) |
28.46 |
\(\ce{CaUO2(CO3)3^{2-} <=> 3 H2O -6 H^+ + Ca^{2+} + 3 CO2(aq) + UO2^{2+}}\) |
22.84 |
\(\ce{Ca2UO2(CO3)3(aq) <=> 3 H2O -6 H^+ + 2 Ca^{2+} + 3 CO2(aq) + UO2^{2}}\) |
19.32 |
\(\ce{MgUO2(CO3)3^{2-} <=> 3 H2O -6 H^+ + 3 CO2(aq) + Mg^{2+} + UO2^{2+}}\) |
23.91 |
\(\ce{UO2OSi(OH)3^+ <=> 2 H2O - H^+ + SiO2(aq) + UO2^{2+}}\) |
2.48 |
Table 2. Surface complexation and cation-exchange reactions
Reaction |
logK (25ºC) |
|---|---|
Surface Complexation (*) |
|
\(\ce{({>}SO)UO2^{+} <=> {>}SOH^{-} - H^{+} + UO2^{2+}}\) |
-0.44 |
Cation Exchange (Gaines-Thomas convention) |
|
\(\ce{Na^+X <=> 1.0 Na^+ + X^-}\) |
1.0 |
\(\ce{Ca^{2+}X <=> Ca^{2+} + 2 X^-}\) |
0.316 |
\(\ce{Al^{3+}X <=> Al^{3+} + 3 X^-}\) |
1.71 |
\(\ce{H^+X <=> H^+ + X^-}\) |
0.025 |
(*) Bulk site concentration = 0.1801 \(\text{moles sites m}^{-3}\)
Table 3. Mineral dissolution/precipitation reactions
Reaction |
logK (25ºC) |
Reference |
|---|---|---|
\(\ce{Quartz <=> SiO2(aq)}\) |
-3.7501 |
|
\(\ce{Kaolinite <=> 5 H2O -6 H^+ +2 Al^{3+} + 2 SiO2(aq)}\) |
7.57 |
[YS08] |
\(\ce{Goethite <=> 2 H2O -3 H^+ + Fe^{3+}}\) |
0.1758 |
|
\(\ce{Schoepite <=> 3 H2O -2 H^+ + UO2^{2+}}\) |
4.8443 |
|
\(\ce{Gibbsite <=> 3 H2O -3 H^+ + Al^{3+}}\) |
7.738 |
[PH95] |
\(\ce{Jurbanite <=> 6 H2O -1 H^+ + Al^{3+} + SO4^{2-}}\) |
-3.8 |
[Nor82] |
\(\ce{Basaluminite <=> 15 H2O -10 H^+ + 4 Al^{3+} + SO4^{2-}}\) |
22.251 |
[Nor82] |
\(\ce{Opal <=> SiO2(aq)}\) |
-3.005 |
[SS00] |
Problem Specification#
Flow and transport#
See the 1D Conservative tracer transport example.
Geochemistry#
Rate expression for mineral dissolution-precipitation reactions
Table 4. Kinetic parameters for mineral reactions
Mineral reaction |
\(log(k_j)\) |
p |
|---|---|---|
\(\ce{Quartz}\) |
-13.345 |
0 |
\(\ce{Kaolinite}\) |
-12.967 |
0.777 |
\(\ce{Goethite}\) |
-7.94 |
0 |
\(\ce{Schoepite}\) |
0.301 |
0 |
\(\ce{Gibbsite}\) |
-11.5 |
0 |
\(\ce{Jurbanite}\) |
-8 |
0 |
\(\ce{Basaluminite}\) |
-8 |
0 |
\(\ce{Opal}\) |
-12.135 |
0 |
Table 5. Chemical composition for the background (initial) and seepage (left boundary) solutions
Component |
Background |
Seepage |
Units |
|---|---|---|---|
\(\ce{pH}\) |
5.4 |
2.5 |
pH units |
\(\ce{Na}\) |
\(2.78\times10^{-4}\) |
\(3.05\times10^{-4}\) |
\(\text{mol kgw}^{-1}\) |
\(\ce{Cl}\) |
\(9.98\times10^{-3}\) |
\(3.39\times10^{-5}\) |
\(\text{mol kgw}^{-1}\) |
\(\ce{CO2(aq)}\) |
\(1.23\times10^{-5}\) |
\(1.07\times10^{-5}\) |
\(\text{mol kgw}^{-1}\) |
\(\ce{Al}\) |
\(2.2\times10^{-8}\) |
\(10^{-8}\) |
\(\text{mol kgw}^{-1}\) |
\(\ce{Fe(III)}\) |
\(2.5\times10^{-16}\) |
\(2.41\times10^{-6}\) |
\(\text{mol kgw}^{-1}\) |
\(\ce{K}\) |
\(3.32\times10^{-5}\) |
\(1.72\times10^{-6}\) |
\(\text{mol kgw}^{-1}\) |
\(\ce{Ca}\) |
\(10^{-5}\) |
\(10^{-5}\) |
\(\text{mol kgw}^{-1}\) |
\(\ce{Mg}\) |
\(5.35\times10^{-3}\) |
\(2.47\times10^{-6}\) |
\(\text{mol kgw}^{-1}\) |
\(\ce{U(VI)}\) |
\(1.25\times10^{-10}\) |
\(3.01\times10^{-5}\) |
\(\text{mol kgw}^{-1}\) |
\(\ce{Nitrate}\) |
\(10^{-3}\) |
\(10^{-2}\) |
\(\text{mol kgw}^{-1}\) |
\(\ce{SO4}\) |
\(2.25\times10^{-5}\) |
\(4.8\times10^{-5}\) |
\(\text{mol kgw}^{-1}\) |
\(\ce{SiO2(aq)}\) |
\(1.77\times10^{-4}\) |
\(1.18\times10^{-4}\) |
\(\text{mol kgw}^{-1}\) |
\(\ce{^3H}\) |
\(10^{-15}\) |
\(2.17\times10^{-9}\) |
\(\text{mol kgw}^{-1}\) |
\(\ce{pCO2(g)}\) |
\(10^{-3.5}\) |
\(10^{-3.5}\) |
\(\text{mol kgw}^{-1}\) |
Table 6. Mineral volume fractions (intial), reactive surface areas and molar volumes
Mineral reaction |
Volume fraction |
Surface area |
Molar Volume |
|---|---|---|---|
\(m^3/m^3\) |
\(cm^2/cm^3\) |
\(cm^3/mol\) |
|
\(\ce{Quartz}\) |
0.88 |
3262.3 |
22.68 |
\(\ce{Kaolinite}\) |
0.11 |
59093.9 |
99.52 |
\(\ce{Goethite}\) |
0.016 |
11076.3 |
20.82 |
\(\ce{Schoepite}\) |
0.0 |
0.1 |
66.08 |
\(\ce{Gibbsite}\) |
0.0 |
0.1 |
31.95 |
\(\ce{Jurbanite}\) |
0.0 |
0.1 |
218.93 |
\(\ce{Basaluminite}\) |
0.0 |
0.1 |
29.0 |
\(\ce{Opal}\) |
0.0 |
0.1 |
126.0 |
Results and Comparison#
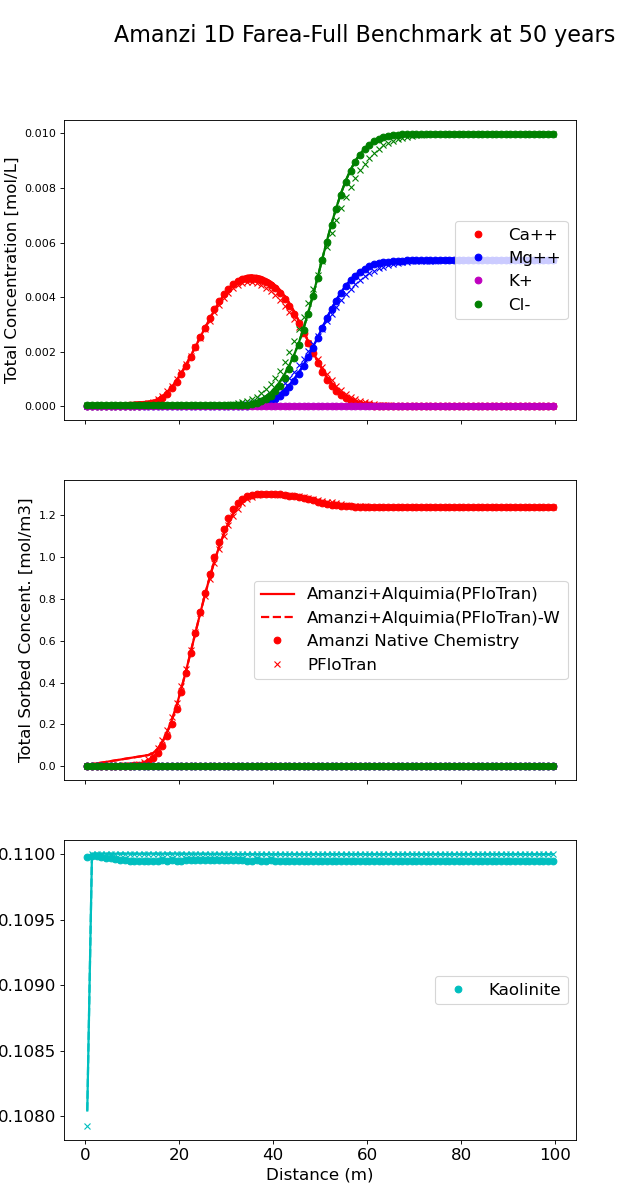
Simulation results#
(Source code, png, hires.png, pdf)
References#
S. A. Bea, H. Wainwright, N. Spycher, B. Faybishenko, S. S. Hubbard, and M. E. Denham. Identifying key controls on the behavior of an acidic-U(VI) plume in the savannah river site using reactive transport modeling. Journal of Contaminant Hydrology, 151:34–54, 2013.
JA Davis, JA Coston, DB Kent, and CC Fuller. Application of the surface complexation concept to complex mineral assemblages. Environ. Sci. Technol, 32(19):2820–2828, 1998.
M. Freshley, S. Hubbard, I. Gorton, J. D. Moulton, C. I. Steefel, V. Freedman, H. Wainwright, and others. Advanced Simulation Capability for Environmental Management (ascem) phase II Demonstration. Technical Report ASCEM-SITE-2012-01 (LANL: LA-UR 12-25496), Office of Environmental Management, United States Department of Energy, Washington, DC, 2012. URL: http://www.osti.gov/bridge/product.biblio.jsp?query_id=1&page=0&osti_id=1055500.
R. Guillaumont, T. Fanghanel, J. Fuger, I. Grenthe, V. Neck, D.A. Palmer, and M. H. Rand. Chemical Thermodynamics 5. OECD Nuclear Energy Agency, Elsevier, Amsterdam, 2003.
M.R. Millings, B.B. Looney, and M.E. Denham. Geochemical modeling of f area seepage basin composition and variability. Technical Report, Savannah River National Laboratory, 2012. URL: http://sti.srs.gov/fulltext/SRNL-STI-2012-00269.pdf.
D. K. Nordstrom. Aqueous pyrite oxidation and the consequent formation of secondary iron minerals. In J. A. Kittrick, D. S. Fanning, and L. R. Hossner, editors, Acid Sulfate Weathering, pages 37–56. Soil Sci. Soc. Am., 1982.
V. A. Pokrovskii and H. C. Helgeson. Thermodynamic properties of aqueous species and the solubilities of minerals at high pressures and temperatures: the system al2o3–h2o–nacl. Am. J. Sci., 295:1255–1342, 1995.
E. Sonnenthal and N. Spycher. Drift-scale coupled processes model, analysis and model report (amr) n0120/u0110. Technical Report, Yucca Mountain Nuclear Waste Disposal Project, Lawrence Berkeley National Laboratory, Berkeley, CA, 2000.
T. J. Wolery and C. F. Jove Colon. Qualification of thermodynamic data for geochemical modeling of mineral–water interactions in dilute systems. Technical Report, Sandia National Laboratory, Las Vegas, Nevada, 2007.
L. Yang and C. I. Steefel. Kaolinite dissolution and precipitation kinetics at 22c and ph 4. Geochimica Cosmochimica Acta, 72(1):99–116, 2008.
About#
Benchmark simulator: PFlotran
Files
Amanzi input file: amanzi-u-1d-farea.xml
Benchmark simulator input file: 1d-farea.in
Location: testing/benchmarking/chemistry/farea_1d
Author: B. Andre, G. Hammond
Testing and Documentation: S. Molins, H.M. Wainright
Last tested on: November 1, 2013
